Page 70 - ITU Journal: Volume 2, No. 1 - Special issue - Propagation modelling for advanced future radio systems - Challenges for a congested radio spectrum

ITU Journal: ICT Discoveries, Vol. 2(1), December 2019

< ( , ) > = 0 ̂
= ( ̂ + ̂) + ̂,
< | ( , )| > = (1)
2
2
ℎ = − ̂ + ̂,
̂
The bracket < > represents the ensemble average
over the space. and
̂ = ( ̂ + ̂) − ̂. (7)
The probability distribution of the surface heights is
constant in space and its correlation function In equations (6) and (7), stands for vertical
( , ′) is given by Equation (2). (parallel or TH) polarization, and ℎ stands for
′
′
( , ) = < ( , ) ( + , + ) > (2) horizontal (perpendicular or TE) polarization.
′
′
′
̂ ̂
The above correlation function is independent of The bi-static scattering coefficient ( , ) along

̂
and . Accordingly, it can be written as ( ) with the scattering direction is the fraction of power

scattered along such a direction with polarization
= √ ′ + ′ . ̂ due to incident wave illuminating the surface
2
2

̂
along the direction with polarization ̂ (Fig. 1).

In the roughness spectral domain , the surface The fraction of power is per unit solid angle and per
correlation function ( ) has a spectral density
function unit area.
2
( ⃗ ) = ( ) ∫ ( ) ⃗⃗ ⋅ ̂ . (3)
2
where is the spatial wavenumber vector. For a
Gaussian single-variant correlation function,
Equation (3) reduces into
1
2 2
( ⃗ ) = 2 {− ℓ }. (4)
4 4
In Equation (4), is the surface correlation length.
For a Gaussian correlation function, the surface
2
height variance and the correlation length may
be used to obtain the surface mean square slope
as in Equation (5).
= 2( /ℓ) (5)
2
2

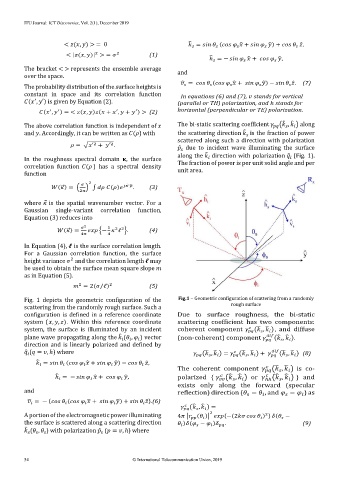
Fig. 1 depicts the geometric configuration of the Fig.1 – Geometric configuration of scattering from a randomly
scattering from the randomly rough surface. Such a rough surface
configuration is defined in a reference coordinate Due to surface roughness, the bi-static
system ( , , ). Within this reference coordinate scattering coefficient has two components:
̂ ̂
system, the surface is illuminated by an incident coherent component ( , ) , and diffuse

̂
plane wave propagating along the ( , ) vector (non-coherent) component ( , ).

̂ ̂

direction and is linearly polarized and defined by
̂ ( = , ℎ) where ( , ) = ( , ) + ( , ) (8)

̂ ̂
̂ ̂
̂ ̂

̂
= ( ̂ + ̂) − ̂,

̂ ̂
The coherent component ( , ) is co-

̂ ̂
̂ ̂
ℎ = − ̂ + ̂,
̂ polarized { ( , ) or ℎℎ ( , ) } and

exists only along the forward (specular
and reflection) direction { = , and = } as

̂ = − { ( ̂ + ̂) + ̂}.(6)

̂ ̂

( , ) =

A portion of the electromagnetic power illuminating 4 | ( )| {−(2 ) } ( −
2
2

the surface is scattered along a scattering direction ) ( − ) . (9)

̂
( , ) with polarization ̂ ( = , ℎ) where

54 © International Telecommunication Union, 2019